 Risk is at the heart of football strategy. Aggressive, risky gameplans should result in boom-or-bust high-variance outcomes, sometimes scoring lots of points but sometimes scoring very few. Conservative gameplans result in relatively consistent low-variance outcomes. Teams would more likely score close to their average score.
Risk is at the heart of football strategy. Aggressive, risky gameplans should result in boom-or-bust high-variance outcomes, sometimes scoring lots of points but sometimes scoring very few. Conservative gameplans result in relatively consistent low-variance outcomes. Teams would more likely score close to their average score.
In this post, I’ll look at what high and low variance strategies would look like in terms of point totals and how they affect each team’s chances of winning. I’ll also compare the theoretical strategies to the actual distributions in the NFL. We'll see why NFL coaches should be more aggressive when they're the underdog.
High Variance Strategy in Basketball
Some time ago, I came across an article posted by basketball researcher Dean Oliver that analyzed high and low variance strategies for the NBA. Oliver calculated the win probability of each opponent according to the mean and standard deviation (SD) of each team’s scoring tendencies. SD represents the degree of variance. The more aggressive and riskier the strategy, the higher the SD will be. For example, a basketball team that shoots lots of 3-pointers would have a high variance.
The key to accurately modeling basketball is realizing that each team’s score is correlated with that of its opponent. The pace of a basketball game ties each team’s score together, and there is a high level of covariance. When one team scores a high number of points, the other team will tend to score more too. Game scores are interdependent.
In Football
Recently the Smart Football blog illustrated the advantage of high variance strategies for underdogs. A high variance strategy increases an underdog’s chances of winning but comes with the cost of also increasing its chances of being blown out.
In the NFL as a whole, visiting teams average about 19 points with a SD of 10 points while home teams average about 23 points with a SD of 10 points. But unlike basketball, football opponent scores are negatively correlated. This makes intuitive sense because the better one team does, the worse the other should do. If one team gets lots of first downs and doesn’t commit turnovers, its opponent will usually start drives with poor field position, and vice versa. The covariance between NFL opponent scores is -1.9 points-squared.
If NFL scores were normally distributed, this is what the typical score distribution would look like. The visitor scores are in red and the home scores are in blue.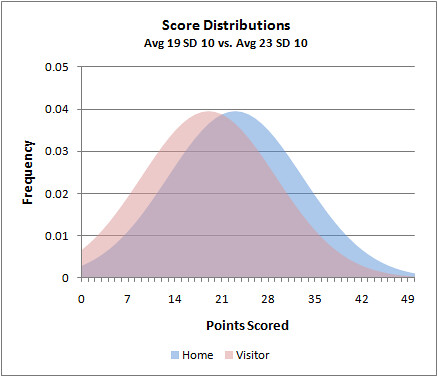
We can calculate each team's chances of winning by summing all the probabilities with these distributions and factor in the covariance using Dean Oliver’s method. This estimates that the home team wins 56.5% of the time, which happens to be exactly the NFL actual home field advantage.
Disclaimer
There’s one problem. NFL scores are not normally distributed, primarily due to its unique scoring, which typically comes in chunks of 3 or 7. Here is what the actual distribution of scores looks like.
The good news is, if we group the scores into bins of 7 points, we get a quasi-normal distribution. (Technically, it may be more of a gamma or Poisson distribution.) I’m going to stick with normal distributions to simplify the math and to better illustrate the concepts I want to convey.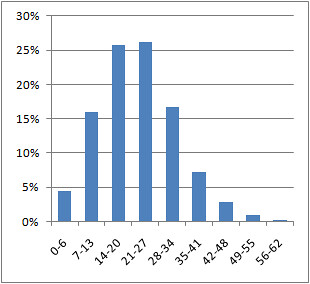
Demonstration
Here’s why underdogs should play aggressive and risky gameplans. Take an example where one team is a 7-point favorite over its underdog opponent. Say the favorite would average 24 points and the underdog would average 17 points. With a SD of 10 points for each team, the underdog upsets the favorite 31.5% of the time. The favorite’s scoring distribution is blue and the underdog’s is red.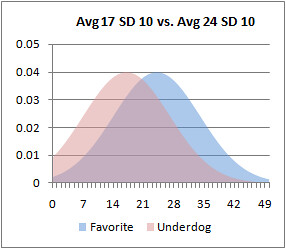
But if the underdog plays a more aggressive high-variance strategy, increasing its SD to 15 points, it would upset the favorite 35.3% of the time.
Note that I haven’t increased the underdog’s average score in any way, just its variance. The increase in its chance of winning results due to more of its probability mass moving to the right of the favorite’s mean score of 24. In fact, the higher the variance, the wider the probability mass will be spread. Consequently, more mass will be to right side of the favorite’s average score. But more mass will also be to the left, meaning there is a higher risk of an embarrassing blowout.
Even if employing a high-variance strategy is non-optimum, it can still help an underdog. In other words, even if an aggressive gameplan results in an overall reduction in average points scored, it often still results in a better chance of winning.
The next graph plots the scoring distributions of just such a scenario. Like before, the favorite’s average score is 24 with a SD of 10. But this time the underdog’s average is reduced from 17 to 16. The increase in variance still results in a slightly better chance of winning despite its overall reduction in average points scored. In this case, it's 33.2% for the underdog.
What about the favorite? Should it increase its variance in response to an aggressive underdog? No. Ideally it should play as consistently as possible. The lower the variance the better for the favorite. The next example shows a favorite playing a low-variance game with an average of 24 points and a SD of 5 points. The underdog is playing conventionally with a 17 point average and 10 point SD. The result is an increase in the favorite’s chances of winning from 69.5% in the original example to 73.0%.
And if the underdog plays an aggressive high-variance game, the low-variance strategy is still better for the favorite. In this case the favorite still improves its chances of winning from 64.7% to 67.8%.
In Practice
So what does any of this mean in the real world? Simply put, to win more often underdogs should employ a high-variance strategy from the beginning of the game. It shouldn’t wait until the 4th quarter and become desperate. Go for it on 4th and short, run trick plays, throw deep, and blitz more often. Roll the dice from the get-go.
The real question is, what is the optimum level of risk? I’m not sure, but I do know NFL coaches are operating far from it.
Looking at games from the ’02 through ’06 seasons (a total of 1280), underdogs do not increase their variance. For example, for games in which the point spread is between 6 and 7.5 points, the underdog’s SD is 9.8 points, slightly less than the overall league average. Ideally, it should be higher. The favorite’s SD is 10.4 points when ideally it should be lower.
The table below lists the SDs of points scored for the favorite and underdog according to the most common point spreads.
| Spread | Favorite SD | Underdog SD |
| 0 - 1.5 | 9.6 | 10.5 |
| 2 - 3.5 | 9.8 | 9.4 |
| 6 - 7.5 | 10.4 | 9.8 |
| 10 - 11.5 | 10.5 | 8.7 |
If anything, there appears to be slight trends in the exactly wrong directions. The bigger the spread, the smaller the underdog’s variance and the bigger the favorite’s variance. It appears underdogs may get less aggressive while favorites may get more aggressive.
Conclusions
This is more evidence coaches do not coach to maximize their team’s chances of winning. My theory is coaches are delaying elimination until the latest point in the game—that is, trying to “stay in the game” for as long as possible. Underdog coaches minimize risk all game long hoping for a miracle along the way. They seem to be reducing the chances of being blown out, but this is not consistent with giving their team the best chance to win.
But if you think about it, this kind of approach might be good for the NFL as a whole. It keeps games entertaining as long as possible, and keeps viewers tuned in.
Coaches of favored teams could be accused of the same crime. They might be playing with too much variance. But there is certainly a limit to just how consistent a team can be, no matter how hard it tries. There will always be random variation in team performance. I suspect a SD of 10 points may be near that limit, and that coaches of both favorites and underdogs simply play the least risky game they can consistent with accepted conventions.








Interesting stuff. As a question, have you looked whether there's any change in SD among underdogs in division games?
It often seems that underdogs are happy to lose by a few points if they don't mind losing to their opponents. No-one wants to lose to a division rival though, and teams may be more willing to risk being blown out in an effort to get the win that no-one thinks you'll get.
Going for it on fourth and short is a statistically sound maneuver based on how easy it is to gain one or two yards. I wonder what would happen to projected outcomes if one team just decided not to punt unless they were buried in their own territory?
More apropos to this post, though, and coaches typically not maximizing their teams' chances of winning any given game, is it possible that this is less that they're stringing the game along, hoping for a mistake, or is it that they're stringing the game along, knowing that a close loss they were never really doing to win anyway is less likely to get them fired than a blowout where they really gave it a good shot?
...never really going to win anyway...
Should then the underdog play the "high variation" strategy until ahead, then becoming the favorite and going conservative?
As you alluded, whether this strategy is sound depends on what happens to the distribution when they switch to "high-variance" mode. I doubt it is safe to assume that the mean does not change, or that it only changes by 1 point. If "high-variance" mode shifts the mean by 3 instead of 1, is it still helpful?
I'm with you on the theory, but I don't think your "in practice" data (on the SD of favorite & underdog scoring) tell us what we need to know, because a team's strategy influences the distribution of their opponent's points scored as well as their own points scored. Risky defensive tactics (like blitzing) directly affect the opponent's offense, and other decisions (like going for it on 4th down instead of punting, or running plays with a higher risk of turnovers) seem like they should influence both teams' scoring distributions.
Mark-no, I don't have division games coded in the database.
Justin-I agree. It's probably all about job security. That's certainly rational, plus fans don't like blowouts either.
JMM-Yup. I suppose if an underdog gets a considerable lead, it's not really the underdog anymore.
drichters-Good point. I don't know the break even point. My examples of SDs of 5, 10, and 15 points are just used to illustrate the concept. In reality, the answer is dependent on how much of an underdog you are, and how an increase in risk will affect your mean score. With some aggressive strategies, like going for it on 4th down, would actually be more optimum and improve the mean score.
Dan-Good point also. Perhaps we really want to know the SD of point difference, not points scored. But defenses don't really have many ways of increasing risk/reward compared with the offenses. I really doubt it's symmetrical like that. Plus, the covariance was -1.9 points-squared, which is actually quite small. So looking at just points scored is going to hopefully be a pretty good indication.
I really liked this post. Well explained, good graphics, empirical evidence ... made my day!
It is nearly 100% about job security which is more valuable than gold in the coaching profession.
Two examples:
Minnesota loses a meaningless bowl game to Texas Tech at the end of December by a score of 44-41. Two days later Glen Mason, Minnesota's football coach, is fired out of the blue. The AD admits it was because of that football game, one in which Minnesota lost a 31-point lead in the 3rd quarter. The RESULT was irrelevant to Mason, who had just signed a long-term extension the year prior. It was the unfolding, or the HOW of that result that got him canned.
Using an NFL example, is Bruce DeHaven, a well-respected coach, fired if the Music City Miracle occurs at any other time in his career? I would guess no. The HOW and the WHEN of the result is what got him canned.
These are obviously extreme examples, but the old canard that it's all about wins and losses just isn't true. HOW you win and lose matters.
Brian, Dean covered this in more depth in Basketball On Paper. Have you read that book?
No, but I take it you recommend it. I'll put it on my list. Can you believe his article that I linked to above has survived online since 1995? Amazing.
Good stuff, Brian.
One thought -- while the covariance between points scored and points allowed may be negative normally, I'm not sure that would remain consistent if the underdog adopted a risky strategy. If they are throwing deep often, that leads to short drives and not taking time off the clock, which gives the opponent more possessions. Similarly, blitzing more often leads to shorter drives.
I agree that coaches in general and underdogs specifically are too conservative, though.
A lognormal distribution is probably best for this exercise given points can't drop below zero.
This is similar to a gambling argument. If you gamble in vegas, you're the underdog. so your best chance is to use your entire bankroll on one bet for a 45% chance of doubling your money, vs. a much lower probability on repeated small bets.
I wonder if coaches applied this strategy historically in the Super Bowl, and that's why we used to get so many blow outs. i.e. I wonder if super bowl scoring differential volatility is higher than the regular season.
Interesting analysis. I think there's more psychology behind current behaviors than just job security, though. For example, if a coach abandons his team's regular game plan / strategy and goes high-risk, he's basically saying "you know what, our usual approach is inadequate." I would think that even a coach who's secure in his job wouldn't want to send that message to his players. Perhaps the "delaying elimination" strategy is actually better for player morale. Players on a bad team might feel better about 3 close losses than it would about 1 lucky win and 2 blowout losses.
With all that being said, it still makes no sense to me that the Lions didn't go no-huddle and run more trick plays once that Thanksgiving blowout put them at 0-12. The D couldn't stop anything, so obviously the O needed to put more than 21 points on the board most weeks to have a chance.
The 2001-04 Steelers make an interesting example on this topic. In '01, they were #1 in rush yds and #21 in pass yds. They lost to the Patriots in the AFC championship game, and Cowher admitted that this loss made him rethink how aggressive his offense should be. In '02 and '03, the Steelers switched from Kordell to Maddox at QB, threw more (~90 attempts/year more), and got worse. After a lousy '03, they put in a rookie QB, threw a lot less, and won the Super Bowl.
Obviously the issues are complicated (did they win because they run more, or did they run more because they won?), but Cowher definitely made the offense more aggressive in 02-03 and less aggressive in 01 and 04, and there's no question that the Steelers were a better team with the more conservative offense. (In this Steelers fan's eyes, anyway)
Steelers fan here also. I'm pretty sure they run when they're winning and pass when they're behind to catch up, but most teams do that.
And not to pick nits, but Roethlisberger went to the AFC Championship (again against the Pats) in his rookie season and won the Lombardi the following year.
steelers fans,
Since they won the championship wouldn't you say they had the superior teams and therefore proved the article right.
Brian,
I was always looking for a way to put into numbers why rivalry games seem to have more unexpected outcomes. Thanks for this article.
Oops, you're right Justin. I should've said that the Steelers won the Super Bowl in 2005.
While it's true that the Steelers, like most teams, run more when they're winning, I really think they tried to throw more in general with Maddox in 2002-2003, and Cowher did say that he wanted to build a more pass-heavy offense during that time. With the arrival of Big Ben, they switched back to a more conservative offense. Whether that was a choice based on the theoretically ideal strategy or a practical one based on having a young QB, I can't say. Probably some of each.
They definitely scaled back when Maddox got hurt because of Ben's inexperience. I could be wrong, but I don't view Cowher as having much of a head for numbers. :)
@parker
Yeah, maybe. I think they definitely had the superior teams in the Super Bowls, but I'm not so sure it was the case in all the playoff games leading up to the championships, especially in 2005.
More to the point, if you go back and watch those playoff games in 2005, Whisenhunt had the Steelers come out throwing the ball, unlike their regular season strategy. If this is an indication of being an underdog (and they were, given they were the 6 seed), then maybe we're on to something for sure.
I seem to remember them being much better than a "normal" sixth seed. They had superior personel and were coming off a 15-1 season.
In order to observe the variance in strategy based on point totals, I'd think you'd have to adjust for the fact that among mismatched teams, the strategies will likely change due to the current score as the game goes on. So it may be that if heavy favorites become more conservative later in the game as they build a lead (and thus underdogs take more risks) the numbers shown in the last table may actually understate the early-game strategic inefficiencies. However, as others have suggested, there might be other in-game strategy changes that affect the results.
Also, while I agree that in theory favorites should be more conservative off the bat and underdogs should take more chances, I don't think anyone has a clue how to modify your team's level of risk-taking without significantly changing your average point-scoring ability. In other words, by increasing its SD to 15 by employing a high-risk strategy, the underdog in your example may be reducing its average points to something so far below 17 that it becomes counterproductive. Recall that the Sackrowitz Chance paper on ball control offense found that the probability of winning is so sensitive to changes in scoring efficiency that underdogs attempting to deviate from optimal pace will likely reduce its ability to score too much to make the strategy worthwhile.
If you somehow defined "conservative" and "risky" decision making, you should be able to show using historical data that underdogs that took more chances did indeed increase their odds of winning (even unintentionally). And vice versa for favorites. But I'd bet that such an effect wouldn't show up empirically because the margin for error is so thin.
And of course, this is all complicated by overwhelming evidence that, when it comes to 4th down decisions anyway, teams don't make optimal choices in neutral situations to begin with.
One thing I find interesting about this research is how, in spite of many coaches inability to go against their own tendencies, it does actually reinforce certain strategic trends.
For example, how often do we hear during a season where a particular team is having an explosive offense that they "should consider running the no-huddle more often" so as to put pressure on the defense, gain favorable mismatches due to limiting personnel/formation adjustments that the defense can make, etc. I would say that this research actually enforces this line of thinking; if one team is across-the-board more talented than another, then increasing the number of opportunities to display that personnel/execution advantage (i.e. run more plays) would logically cause each team to move more towards its performance mean if you will.
So, in that, I think it is wise for an underdog to almost NEVER run a no-huddle offense (unless their offense is considered better than the other teams defense). With that said, the same said offense should then, as you said Brian, throw deep more often, run trick plays, perhaps use more unusual formations/motions/formation shifts, etc. but that the actual tempo of the game should be fairly slow.
I would be interested to look at teams during what would be deemed a dynasty era that "overachieved" early in their dynasty run (and see how their use of the no-huddle compared with that of their more dominant years later on. I am willing to beat we would see a faster tempo from the more talented team than the early team.
For example, the 2001 Patriots were, by most standards, considered a team that had mediocre, 8-8 type talent on their roster that went on to win a Super Bowl. I would be willing to beat that the 2007, 16-0 Patriots who were eventually upset by the N.Y. Giants ran the no-huddle significantly more than did the 2001 Patriots...ditto the 49ers of 1989-1990 versus 1981 (first championship of that dynasty, also won by a team considered not overly talented).
As both Bill Walsh understood and Bill Belichick understand the value of analysis of tendencies (both their own and the opposition's) and metrics in determining strategy, I bet both dynasties would provide interesting support for the no-huddle variance strategy I am speaking of.
Michael-I agree. That was my recommendation for the Giants in the SB 2 yrs ago. The more iterations in a contest, the more likely the better opponent will come out on top.
Underdogs and Reducing PossessionsSuper Bowl XLII and Team Possessions
Although the high-variance strategy produces wins more often for the underdog, doesn't it also produce more heavy losses?
Coaches might be worried about the morale effects on the players of a heavy loss (and not just the effect on the fans and the owners!)
The 2008 Arizona Cardinals are a good example of a team successfully following the strategies dictated in this column. Against their lousy divisional foes, the Cards were much more conservative than against the non-divisional opponents. They suffered numerous blowouts which caused pundits to see them as less capable of defeating elite teams than they actually were. In fact, their high risk/reward strategy prepared them beautifully for the playoffs where you must defeat a number of elite teams consecutively.
This is where teams like Baltimore and Tennessee tend to struggle. Their conservative approaches, which work so well during the regular season where most of their opponents are inferior, do not prepare them for the playoffs. The Steelers appear to be a similar team, but their QB/WR situation is much better suited for the risks often necessary in the playoffs.
Part of what makes the NFL so interesting also makes drawing conclusions from articles like this difficult. There are so many moving parts. However, it is worth noting that sometimes increased risk can dovetail with apparently counterintuitive strategies like slowing the pace. When an underdog goes for it on fourth down, that is in apparently risky strategy, but, when successfully executed not only allows them to retain possession but also succeeds in reducing overall possessions.
Brian,
excellent column. I found your blog about a month ago and must say it is well-written.
As a long-time Madden and NCAA gameplayer, I've employed an underdog strategy of playing 4 downs every time past my own 45, calling lots of trick plays while using every second of the play clock every down. This has increased my chances of winning. Completely unofficial, but possibly interesting as an anecdote.
In the "In Practice" section, you note that the favorites tend to have a higher SD, when you'd expect them to play a lower-variance strategy. I wonder if this is related to the general fact that coaches seem to be too conservative on things like 4th down.
If you've established that coaches tend to be too conservative on 4th down calls, then better coaches will be more aggressive than average on those calls. This will make them win more games, and therefore be favorites more often, and it will increase their variance as well.
I may be missing something, but given that big favorites are expected to score a lot of points and big underdogs are expect to score very few points, shouldn't the Standard Deviation of big favorites naturally be higher than the SD of big dogs due to the level of the mean? For example, if a big favorite is expected to score 28 points, while a big underdog is expected to score only 10 points, shouldn't the SD around 28 be higher than the SD around 10 simply because 28 is much higher number than 10?
I concede there could be a very simple explanation that I do not understand. Please help me out if that is the case.
I ran the following with the data I have:
When the favorite is a 10-point or greater favorite, their SD was 10.2 with an average of 27.1 points scored.
When the favorite is a 3-point or less favorite, their SD was 10.1 with an average of 21.4 point scored.
When the underdog is a 10-point or greater underdog, their SD was 9.7 with an average of 19.5 points scored.
This is using data from the last 20 years.
I do not know if it is accurate to divide SD into average points, but if I did that, I'd get:
Fave of 10+ 37.7%
Fave of 3- 47.1%
Underdog of 10+ 59.2%
Underdog of 3- 49.8%
If this is an accurate way to look at it, then it seems that big underdogs may be playing with more volatility than big favorites.
Again, I concede I am missing something or I am not making a correct interpretation or doing a bad statistical leap...so please correct me when I'm wrong.
P.S. What years were your data from?
Quick question based on yesterdays Cowboys/Chiefs game - what do your statistics say to you about going for the two point conversion versus kicking the extra point to tie the game (as the chiefs did with :24 remaining). I was somewhat surprised they didn't go for it, especially considering they were 0-3 at the time and a significant underdog. So my question incorporates the general statistical advantage of playing for overtime at home (questionable at best) versus the straight statistical probability that the offense can gain two yards on 4th down. Given the complexity of probabilities in playing for the tie and the dependency of those probabilities on outcomes not under your control (coin-flip), wouldn't ANY team be better off in this instance simply attempting the two point conversion? Or am I simply too overaggressive?
With all due respect, I'm not sure that I buy some of the conclusions in this article. As others have noted, there are two components at work here when we talk about overall point variance: the variance of points in a possession, and variance in the number of possessions in a game. It seems that strategic decisions will primarily affect the latter category, and if we assume that higher-risk strategies tend to produce more possessions per game, then this works to the detriment of the underdog. (The converse of this is yet another reason why New England's refusal to go no-huddle in the SB was so baffling.)
Unless I'm missing something, it doesn't really make sense to speak of a team deliberately raising their standard deviation of points per possession, with the specific exception of strategies that will minimize the probability of a field goal without affecting overall point expectation. With that in mind, I certainly agree that underdogs should be more inclined to play for a touchdown instead of a field goal when it comes to fourth-down decisions, and play-calling near field goal range in general. But what does an underdog gain by blitzing and throwing deep? Either these plays increase point expectation, in which case they should be doing them anyway, or they will merely tend to raise the number of possessions in the game, in which case they are playing into the hands of the opponent.
In other words, point expectation on an offensive or defensive possession is based on the underlying 0/3/7 score probabilities. Much as it seems to make some intuitive sense that ostensibly risky strategies such as blitzing and throwing deep should simultaneously raise the probability of scoring/allowing either 0 or 7 points on a possession, this doesn't make sense unless there's a compensating decrease in the probability of 3. While this may indeed be the result of such strategies to some degree, it seems as if it would apply disproportionately to play selections near the edge of field goal range. And these strategies still suffer the drawback of tending to increase the number of possessions, so it's really not clear to me that their use tends to benefit the underdog at all. In any case, I think it should be made clear in the article that the prescription applies primarily to this region of the field. Otherwise, it looks as if we're just superposing mathematical models onto the question without considering how they actually interact with football reality.
I guess the other thought process to take into account is that these strategies might be affecting the transition probabilities: we take a bigger risk of incurring a field position deficit - thereby increasing the opponent's probability of scoring - in order to raise the probability of scoring ourselves. But I'm not sure that this really makes a difference. We're operating under the hypothesis that expected net points are not changing as a result of this strategy, and this is ultimately comes back to the 0/3/7 probabilities on offense and defense. Maybe the key intuition is that a given net point differential is less significant, in terms of pythagorean win probability, as the total number of points increases, so it makes some degree of sense for an underdog to aim in this direction. Though I still think this runs into the objection that increasing the number of possessions, when facing an expected deficit per possession, can never be a good thing.
I know I'm kind of thinking out loud here, but I'm really trying to understand why this conclusion would hold true, and not quite getting it yet.
In a year when the NFL is being dominated 300-400 yards through the air a game, with the #1, currently 12-0 team in the nfl having the league lowest rushing totals (though also tied for the league's 2nd most rushing points), while some of the bottom-feeders like the Raiders seem to do nothing, and lose (except to the steelers o.O), and dothers like the Rams and the Bills try to throw the ball and be aggressive all day get nowhere...
I think we have ourselves an anomaly =]
Or...The points here aren't as accurate as they initially seem.
Oh sure, I could see looking at your avg/rush, distance to 4th down, and 4th down conversion rate and realizing it'd be prudent to go for it a few times you'd have never thought of it before, however...
This entire article Seems to indicate that Aggression in a game creates scoring and Conservatism limits it on both sides of the ball.
I would suggest that "aggression" is probably not going to win an underdog a game, in fact, if they are the less talented game, it will probably just make it worse.
For example, Let's look at the Lions and the Colts. Colts would be a huge favourite, so according to this article, the right thing to do would be for the Lions to play as aggressively as possible, passing deep, avoiding burning the clock, so maybe go no huddle, don't really run the ball if you can help it, except in very short yardage situations, and trust your defense to keep up as you keep them on the field for half a quarter longer than your opponent.
Further, in order to safeguard themselves, the Colts should play conservatively. Huddling between run plays to run the clock, and not putting pressure on the lions qb, favoring zone coverages instead.
Ideally, that should work, right? Except, this article completely ignores the dynamic matchups that decide NFL games. The Colts have the #1 passing game with the Last ranked running game, while the Lions are bottom last at defending against the pass, and are in the bottom quarter of the league for passing it themselves, combined with their inability to convert on 3rd down, and an even worse 4th down percentage, and 10 giveaways in 12 games, I really don't think they'd be giving themselves any chance whatsoever to win this game, but the Colts would also be inexplicably toning down what they do best in favour of what they do worst.
Of course, this is about as an extreme of a contrast as you can get between two teams, but that's why I used it to illustrate the one-dimensionalness of "agression" vs "conservatism" as defining strategies.
Instead, it's about getting the edge on a mismatch and exploiting it, that's what wins games. So for an upset to occur, the underdog has to find an unexpected exploit, and push it all they can, and hope it's enough. Sometimes, sure, it'll be a hole in their coverage zones that throwing it deep will exploit, or it'll be a bad matchup on the corner with your RE, and you'll play more aggressively on both sides of the ball. Or maybe, it'll be that the Colts' run efense is in the bottom half, and the Lions can chip away at them by running it up the middle. Would it be enough to win? Probably not, but the point is, throwing the game plan away straight away in favour of some ideal is *not* going to work. You have to stick in it as long as you can and keep hoping to find that edge that'll prove you're /not/ the underdog in the matchup, or to realize that you were called the underdog for a reason, and you're going to lose.
=]
What most people don't know, but should is that football is like a coin that has two sides. Everyone knows the universal truth of the first side of the coin that says that a team that scores more points than the opponent always wins. What people don't know is how a team lost a game without factoring the score. The other side of the coin would say that the team with the most real turnovers always losses the game. Statisticians do not count all events that turn the ball over without first scoring atleast 6 points. If they did they would see that the team with the higher number always losses. Try this with any game and you will see that it works:
Complete Turnovers: Interceptions, fumbles lost, missed fg's, punts, 4th down fails, kickoffs lost, safeties, and turnovers due to time (given if the team in possesion at the end of the half failed to score and has to kick to start the second half. Not counted if another turnover half or whole occurs at the same time. Also when the game ends and the team trailing fails to score before the time expiresn not counted against the team with the lead. also nullified if another turnover takes place.
Half Turnovers: Failed extra point or 2pat, Successful field goal, 2point conversion allowed.
Yes...with the touchdown being worth 7 and the field goal only 3, it's as if the only time the ball should be punted/kicked is if 1) you are not past the 50 yard line, go ahead and punt you don't want to give your opponent the ball & already in position to strike with a 20 yard pass 2)you are beyond the 50 but it is a 4th and 8 or more say..you should always have a play that you can be confident can get you about 7 yards maybe so if you don't get it fine...you are still leaving your opponent in bad shape, but if you get it!, then you still have an opportunity for SEVEN...or 3) it's the end of the game and the score is 26-24 and there are :04 seconds left and you can go ahead and send you FG kicker out to win it. AKA you need 3.
I believe this would be just as effective scoring as the way teams play it now...and a more exciting brand of football.
Really, it's interesting to think that if you basically forced the issue with the touchdown and punted when it was wise to do so...you'd just have to get in the endzone 4 times and you've got 28...4 touchdowns beats 9 field goals in the game of pro football, basically. Or more realistically, let's say 4 touchdowns vs. 2 touchdowns & 4 field goals. 28-26.
Sometimes to kick the 3 would avoid a turnover and go ahead and get your team 3 points. It's not as if converting on 4th down guarantees 7. It could result in an interception run back by the other team, or another loss on downs if you try forcing the issue and going for it on 4th down again. Putting 3 on the board for your team ain't a bad idea if you got a kicker you can really trust and you're really not particularly confident about getting in the endzone on a particular drive against certain defenses especially and always depending on the score and the time.
"The bigger the spread, the smaller the underdog’s variance and the bigger the favorite’s variance. It appears underdogs may get less aggressive while favorites may get more aggressive."
You only listed SD in the favorite and underdog's scores, not the means. Presumably the means are higher for the favorites.
So looked at relative to the mean scores, maybe favorites and underdogs are the same, or maybe the trend even goes in the "right" direction?
Coaches do not want to be aggressive unti.l the end,,, because if they are aggressive earlier there is a counter adjustment. Problem with game theory and Nash equilibrium is with a low sample size people are fooled by randomness. In reality there is a lag time towards adjusting... Thus higher care piece towards end means opponent can't adjust.
If you have a really good play against a defense and can keep the game within a TD that play the first time you try it might work 80% of time. Opposing team will then be able to counter on offense with re,wining time but if you already have tried play the better team will get aggressive if need be, gain a lead and counter your strategy. After you have tried a trick play or carefully designed play its success rate may drop to 60% and then 40%. Timing in when you use effective plays is important.
I would expect SD to be lower for teams who are bigger underdogs simply because I expect them to score less. I doubt it has anything to do with their strategy. If you were to look at college games with huge spreads, my guess is the SD is even lower, because the teams never score.
jim campasano--
Good point. You might be interested in the Coefficient of Variation, where the standard deviation is divided by the mean to get a "unitless" measure of volatility:
CV = SD/mean
http://en.wikipedia.org/wiki/Coefficient_of_variation
In fact this is what Anonymous calculates above--see September 17, 2009. (Note: I think there is an error in Anon's calculations: "Underdog of 10+ 59.2%"; it seems to me that the percentage should be 9.7/19.5 = 49.7%. Also, there appears to be rounding error on a couple calculations; I suspect that Anon is calculating with more significant digits in the mean and SD than he/she posts.)
It's worth pointing out that Anon's numbers are very similar to the author's: SD's of a little more than 10 points for big favorites, and a little less than 10 for underdogs. Anyway, Anon points out that there does appear to be a slight trend toward higher CV's for bigger underdogs (the underdogs have smaller SD's, but they have smaller means also).
However, does this indicate a difference in strategy between the two populations? No; there may be many explanations for the apparent difference in CV's, not least of which is that the same level of randomness would necessarily have a greater relative effect on a team with a smaller mean. (The difference between a touchdown and a field goal is a big RELATIVE effect if your mean is 14; not as much of a relative effect if your mean is 35.)
Upon reflection, it's not clear to me that either the CV or the SD is really a good way to measure a team's strategy. However, I do agree with the author and with several individuals above that underdogs should attempt to do two things:
1) Limit the total number of possessions (to increase the effect of randomness). Since the clock is fixed, this is equivalent to increasing the average time off the clock per drive.
2) Adopt a high-risk, high-reward strategy (again, to give randomness an increased role in the game's outcome).
Unfortunately, it is hard to think of strategies that do both. For instance, throwing deep is a high-risk, high-reward strategy that amounts to throwing the dice and betting a lot on the outcome of a single play, so it may be a very good approach to Goal 2, but it is a very poor approach to Goal 1: deep passes tend yield a lot of incompletions, which stop the clock, as well as sacks, interceptions, and quick touchdowns, none of which will tend to achieve Goal 1. (Note: obviously quick touchdowns are great...but by definition they do not take much time off the clock.)
For the favorite also, it's hard to come up with strategies that achieve the OPPOSITE of both goals: conservative, low-risk play-calling means runs and short passes, but these tend to result in long, grinding drives and lots of time of possession (which results in fewer possessions per game, exactly what the favorite does NOT want).
However, there is room for a few observations. A favorite can benefit from running a no-huddle hurry-up offense most of the time...assuming that their offense will be just as effective without huddling (a big assumption). And a weaker team should generally take as much time off the clock between downs as possible. Also, as a lot of others have already pointed out, an underdog should be willing to go for it on 4th down in a lot of cases; this type of strategy could help to accomplish both goals.
Often an underdog can grind it out, make it a low-scoring game, and hang around within striking distance of the lead for the first three quarters or more, and then bet the whole game on a single drive at the end. In many cases, that may be the best they can hope for. If so, this might give an alternative explanation for why an underdog would play conservatively until the end: maybe it's not that they're irrationally ignoring Goal 2, but are rationally pursuing Goal 1 (this strategy would be rational to the extent that Goal 1 was deemed more important than Goal 2, which has yet to be determined).