So assuming that a defender that beats his blocker would always sack the quarterback, the blocker should hold him whenever he believes the probability of detection is lower than about 0.81. In other words, he'd get away with it 1 out of 5 times. It's understandable why a blocker would intentionally hold a pass rusher in this situation. The bottom line is that the probability of detection at which committing holding is worthwhile is when it is about 4/5 the chance a pass rusher will get a sack if he beats his blocker. For argument's sake, say that a pass rusher in the backfield gets a sack half the time. The probability of detection would need to be below 0.4 for the hold to make sense. It all boils down to the graph below: So all a blocker needs to do is quickly solve the equation above immediately after the snap, given his estimate of...I'm just kidding. Of course I don't expect anyone to use math to make decisions in the heat of battle, but this analysis does explain one reason why we see so much holding. There are other complicating considerations too. A pass rusher could miss the sack but hurry the pass, causing an incompletion or worse. There are all kinds of possibilities. But ultimately, despite the apparent harshness of the penalty, the infraction is not always called, and in many cases can be worth the cost. Nothing upsets a coach more than a offensive holding call in the middle of an otherwise productive drive. The play, usually a good one, is nullified, and the penalty moves the line of scrimmage back 10 yards. What was a promising 2nd and 5 becomes a difficult 2nd and 15.
Nothing upsets a coach more than a offensive holding call in the middle of an otherwise productive drive. The play, usually a good one, is nullified, and the penalty moves the line of scrimmage back 10 yards. What was a promising 2nd and 5 becomes a difficult 2nd and 15.
Yet holding calls are frequent, which suggests there's obviously something useful about holding. For passing plays, the alternative is often a sack, which is bad in all kinds of ways. Plus, not all instances of holding are called. I'm sure if you polled defensive lineman, they'd say less than 10% of holds are actually flagged.
So I wanted to know, "for passing plays, what's the break-even detection rate for a hold which would make it worthwhile?"
It's a complex question with lots of variables, so let's isolate some. First, let's define the utility of "worthwhile" as based on the probability of converting a first down. Consider a general 2nd down and 5 situation. Typically, an offense in that situation that calls a pass will convert for a 1st down 71% of the time. We'll note this as P1D = 0.71.
An offensive holding penalty negates the play and penalizes 10 yards from the previous spot, forcing a 2nd and 15. That makes the chances of a 1st down considerably lower. The probability of a 1st down "given a hold" is P1D|Hold = 0.20. For all 2nd and 5 pass plays in which there was no sack, the probability of conversion is P1D|NoSack = 0.73. But for all 2nd and 5 plays that resulted in a sack, the probability of conversion is P1D|Sack = 0.30.
For all 2nd and 5 pass plays in which there was no sack, the probability of conversion is P1D|NoSack = 0.73. But for all 2nd and 5 plays that resulted in a sack, the probability of conversion is P1D|Sack = 0.30.
In order of preference, you'd rather have neither a sack nor a hold (0.71), then a sack (0.30), and lastly a hold (0.20). But not all holds are called. I'm not sure what the detection rate really is, but we can solve for what detection rate would make a hold worthwhile.
For now, let's assume that if the pass rusher beats his blocker, he will cause a sack 100% of the time. And let's call the ref's holding detection rate "x." The break-even detection rate could be found with a simple linear equation:
Solving for x, we get:
.20(x) + .73 - .73(x) = .30
-.53(x) = -.43
x = .81
But pass rushers who beat their blockers don't sack the QB 100% of the time, so let's generalize the equation. Call the probability of a sack given the defender beats his blocker "y." The break-even equation now becomes:
Simplifying, we get:
.20(x) + .73 - .73(x) = .30(y) + .73 - .73(y)
-.53(x) = -.43(y)
x = .81(y)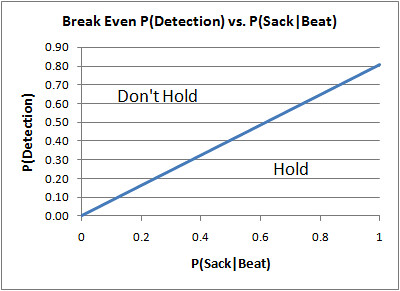
Note: Data is from the 1st quarter of all NFL games 2000-2008. Other quarters are excluded to eliminate the effect of "end-game" plays--hurried plays at the end of the halves, desperation plays by trailing teams, and clock-burning plays by leading teams.
Why There Is So Much Holding
By
Brian Burke
P1D|Hold(x) + P1D|NoSack(1-x) = P1D|Sack
.20(x) + .73(1-x) = .30
P1D|Hold(x) + P1D|NoSack(1-x) = P1D|Sack(y) + P1D|NoSack(1-y)
.20(x) + .73(1-x) = .30(y) + .73(1-y)
Subscribe to:
Post Comments (Atom)








I am amazed that a 2nd and 5 play that results in a sack, yielding, say, 3rd and 12, is more likely to lead to a first down than a 2nd and 5 play that results in a hold, yielding 2nd and 15. I guess there must be significant fraction of sacks where the QB manages to get back to near the line of scrimmage before going down. Can you tell us the average yardage-to-go after a sack on 2nd and 5?
Will-This sack article should answer your question.
I'm in the same boat as Will -- even after reading Anon's sack article link. Dropping 10 yards on the 2nd down line in the 1st down probability graph looks to be about a 30% drop -- same as the prose talks about for a sack. But, the sack also has the increased fumble rate to contend that should make it less likely to get a first down.
Sacks cause a about a 5-yd loss on average, and that holds across the spectrum of downs and to go distances, including 2nd and 5 (-5.18 yds). Hope that helps clarify things. I double checked the numbers to be sure.
Yeah -- that's what your article says too. A sack is equivalent to a penalty that incurs 5yrds and a loss of down; whereas a hold is 10 yards. The article (and graph) indicate that the the sack reduces the 1st down prob by 30% -- which looks to be similar to a 10 yard difference along the same down line. It also talks about an extra expected point loss because of the enhanced risk of fumble. (Was the fumble risk included in this study?) All said, its very surprising that a sack is 10% better than a hold
Yes, it factors in fumble risk, but only in as far as it affects the probability of gaining a first down. The consequence of a lost fumble is only partially considered here.
Do mobile QB's, or those that are adept at getting rid of the ball (e.g. Manning), result in OL's instinctively knowing that the probability of sack is lower and therefore they hold less?
Conversely, I'd imagine mobile QBs (especially, out-of-the-pocket guys) result in more holds. Defenders moving laterally rather than running down-hill into the pocket are probably tougher to legally block.
When protecting a running QB, the rushers nearest to the passer are harder to block, but the rushers he is rolling away from are easier to block. By eliminating half the rushers you should at least eliminate the holding penalties on them. I'd say the conventional wisdom among most coaches is that putting the QB on the move makes him easier to protect. This is a testable hypothesis, but as far as testing whether a mobile QB in general is easier to protect, we'd first have to quantify the meaning of "mobile."