 Should a team that adds a superstar running back run more often? It seems like a dumb question, but the answer isn't so simple.
Should a team that adds a superstar running back run more often? It seems like a dumb question, but the answer isn't so simple.
In 2006 the Minnesota Vikings ran the ball 388 times for 1820 yards, a 4.1 yard per attempt average. In 2007 the Vikings added rookie sensation Adrian Peterson who helped the Vikings run 440 times for 2634, a 5.3 yard per attempt average. That's an increase of 52 runs from '06 to '07, a 13% increase. (Rush attempt figures do not include QB runs or kneel-downs).
Part of the increase might have been due to the Vikings ' two more wins in '07 than in '06. Since running is a safer option late in games with leads, we could expect a few additional run attempts, but certainly not 52. With the addition of a star RB like Peterson, should a team like the Vikings have run even more? Or did they run too much?
Conventional wisdom might say of course. Why wouldn't a team use its biggest advantage more frequently? But think about it from the point of view of an opposing defense. They know how big a threat Peterson is, and would likely orient their strategy toward stopping the run. Knowing this, what should the offense do? There are 3 options:
1. Run more often than before because every run will bring an increased payoff.
2. Pass more often because the defense will be stacking the line with run defenders.
3. Run the same proportion as before. Considerations 1 and 2 balance out, and payoffs will increase for both passing and running.
From my recent article of game theory and strategy, recall this simple payoff matrix (below). An offensive run play against a run defense will usually result in a setback. But against a pass defense it will be more successful. Similarly, a pass against a run defense is likely to be more successful. The payoffs don't necessarily represent yards or points, but rather a proportional preference for the various expected outcomes.Before star RB Defense Run D Pass D Blitz Offense Run -3 4 6 Pass 9 -3 -5
Now let's add a star RB to our team. Let's say our expected run outcomes increase by 1 full unit against each defensive strategy. Our passing outcomes remain unchanged. The new payoff matrix looks like this:With star RB Defense Run D Pass D Blitz Offense Run -2 5 7 Pass 9 -3 -5
How would this change affect the optimum run/pass balance for the offense? The original payoff matrix is illustrated in the chart below. The x-axis represents the run/pass mix of the offense. The left side of the chart indicates the payoffs vs. the defensive strategies if the offense runs 100% of the time, and the right side of the chart indicates the payoffs if the offense passes 100% of the time. In between are the payoffs given various proportions of running and passing.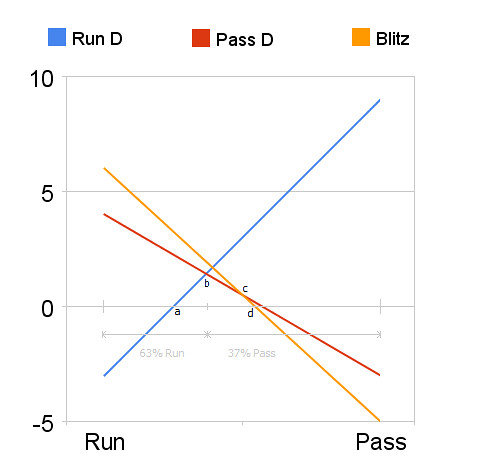 The optimum play mix, where the payoff to the offense is maximized, is the intersection of the red and blue lines at point b. In the example (before adding the star RB), the offense should run 63% of the time and pass 37%. As long as the offense optimizes, the blitz (orange) strategy is not part of the defense's optimum strategy mix. We can ignore it for now.
The optimum play mix, where the payoff to the offense is maximized, is the intersection of the red and blue lines at point b. In the example (before adding the star RB), the offense should run 63% of the time and pass 37%. As long as the offense optimizes, the blitz (orange) strategy is not part of the defense's optimum strategy mix. We can ignore it for now.
Graphically, when an offense's run performance increases, the payoffs on the left side of the chart move upward. The payoffs on the right side of the chart (the passing payoffs) stay fixed. In effect, we get a hinged upward swing of all the payoff functions. As run performance increases, notice what happens to the optimum play mix (the intersections). The optimum ratio of running and passing remains constant. Running yields bigger payoffs, but defenses would have to adjust, allowing the passing game to face run defenses more often. In the example where we added 1 unit of payoff to every run play, the intersection is where yRUN D= yPASS D. Therefore:
The optimum ratio of running and passing remains constant. Running yields bigger payoffs, but defenses would have to adjust, allowing the passing game to face run defenses more often. In the example where we added 1 unit of payoff to every run play, the intersection is where yRUN D= yPASS D. Therefore:
-2 + 11x = 5 -8x
19x = 7
x = 7/19 = 0.37
Did the Vikings run too often? Who knows. In theory, all things being equal, the addition of a superstar RB shouldn't alter an offense's play selection. The passing game should open up, and the offense will improve overall. In fact, that's what happened to the Vikings between '06 and '07. With a very shaky Tavaris Jackson under center, they still improved from 5.4 net yards per pass attempt to 5.8 after adding Peterson.
So if the relative strengths of the offense doesn't affect the theoretical optimum run/pass balance, what does? Increasing pass performance would just lead to the same balance. The graph would be hinged on the left instead of the right, but the optimum proportion would remain constant. The result is the same when the defense improves or worsens. Oddly, the only way to achieve a shift of the optimum run/pass balance is for either or both offensive strategies to improve against one of the opponent strategies but not the other. For example, the optimum balance would shift toward the pass if an offense improved both their run and pass performance against a run defense without improving against the pass defense. Confused? Perhaps you should be--such a scenario would be hard to imagine. How would a team's running or passing improve against a run defense but not a pass defense?
The result is the same when the defense improves or worsens. Oddly, the only way to achieve a shift of the optimum run/pass balance is for either or both offensive strategies to improve against one of the opponent strategies but not the other. For example, the optimum balance would shift toward the pass if an offense improved both their run and pass performance against a run defense without improving against the pass defense. Confused? Perhaps you should be--such a scenario would be hard to imagine. How would a team's running or passing improve against a run defense but not a pass defense?
Admittedly, this is a highly theoretical analysis. In reality play selection has much more to do with game situations than anything else. But the analysis does explain why teams with apparently vastly different passing and running abilities all tend to share similar run/pass proportions. With rare exceptions, teams stick very closely to the NFL average 45% run proportion regardless of how their running game compares to their passing game.
Game Theory and Great Running Backs
By
Brian Burke
published on 6/20/2008
in
game theory,
research,
run-pass balance,
strategy
Subscribe to:
Post Comments (Atom)








"With rare exceptions, teams stick very closely to the NFL average 45% run proportion regardless of how their running game compares to their passing game." That's great info, and I think you're right on the money as to why that is (whether or not coaches are consciously aware of it). If only this was the type of analysis we see on Fox NFL Sunday.
I just want to clarify that I'm not claiming that the actual optimum ratio is 63% run/37% pass. It's just an example for demonstrating how game theory would solve the issue using notional numbers.
The actual solution would be more complex, and would there would be different solutions to different down and distance situations. The optimum ratio on 1st and 10 would obviously be different than on 3rd and 8.
This is great stuff, both this post and the previous one. (As a side note I am now linking up your blog to mine. Hopefully I post with more frequency.)
On this topic I'm trying to think of what might happen if you had teams that were, in some abstract sense, already heavily skewed. I'm thinking of say a wishbone type team or a pass-happy college team like Texas Tech. I suppose that would just change the initial equilibrium because the payout for throwing against a run D might be higher (say 15 instead of 9, but the run payout against a pass D might be lower). I don't have my number crunchers in front of me, but is the upshot here that a team has a rough initial equilibrium but would move little after that, without a wholesale change? I hope I'm not misreading it.
In any event, I just wanted to mention a point I've been thinking about for some time, highlighted by Alamar's and Rockerbie's papers. I think strict average yardage maximization breaks down in some football situations.
For example, most 3rd downs. This might be simplistic, but I would chart (almost) all 3rd downs as binary: you either got it or you didn't. Now obviously sometimes on 3rd and 1 from midfield the offense breaks it for a long TD run or even runs a play action pass going for it, knowing that their percentage of success isn't great but that if they do it is a TD, but it will skew your statistics to factor in all the 3rd and short and 2nd and 2 runs into the same yardage totals problems. (No one thinks taking a knee should skew you back to the passing idea.)
One of the difficulties is you need to practically be on an NFL staff to gather this kind of info, but it's interesting because as significant as this work is (and it is), it's worth keeping in mind these other scenarios. That said, the vast majority of football plays take place on 1st and 10, and the analysis obviously holds true then. (Or should!)
You state that the NFL's normal rush to pass ratio is about 45% rush, but in your example you use 63% and qualify it by stating it is, "an example for demonstrating how game theory would solve the issue using notional numbers."
Have you run the real numbers, in particular for the Vikings and do they come out closer to the league average of 45% or the actual percentage that coach Childress/Bevell called running plays?
Nice blog. I enjoy the science of the game and stats analysis breaks that down. Advanced NFL Stats has just been added to my reading list.
Luft-No I haven't run the actual numbers. There are some issues to solve first. You'd have to have a lot of good data of rushes when the defense knows the play will be a rush, and of passes when the defense knows it will be a pass. Plus, we'd have to have a separate analysis for each down and distance situation. Lastly, we'd also need a valid utility function, because yards do not = utility.
well put it like this adrian needs to get the ball more on 3rd and jackson needs ta scramble more
This is an excellent post. It explains the football cliche that you need to make them respect the run. passes are better than runs but if you pass every time they will just run a pass defense every time and you are SOL.
Really interesting post. However, I think there is a mistake in the paragraph about the only way to cause a shift in the optimum run/pass balance.
You write that, "For example, the optimum balance would shift toward the pass if an offense improved both their run and pass performance against a run defense without improving against the pass defense."
In that case, wouldn't the optimum balance shift toward the run? This is because more running plays would result in the defense playing more run defense. Since the offense has improved both the run and the pass against the run defense, the balance should shift toward run.